In algebra, a decimal number can be defined as a number whose whole number part and the fractional part is separated by a decimal point. The dot in a decimal number is called a decimal point. The digits following the decimal point show a value smaller than one.
Fractions represent equal parts of a whole or a collection. A fraction has two parts. The number on the top of the line is called the numerator. It tells how many equal parts of the whole or collection are taken. The number below the line is called the denominator. It shows the total divisible number of equal parts the whole into or the total number of equal parts which are there in a collection.
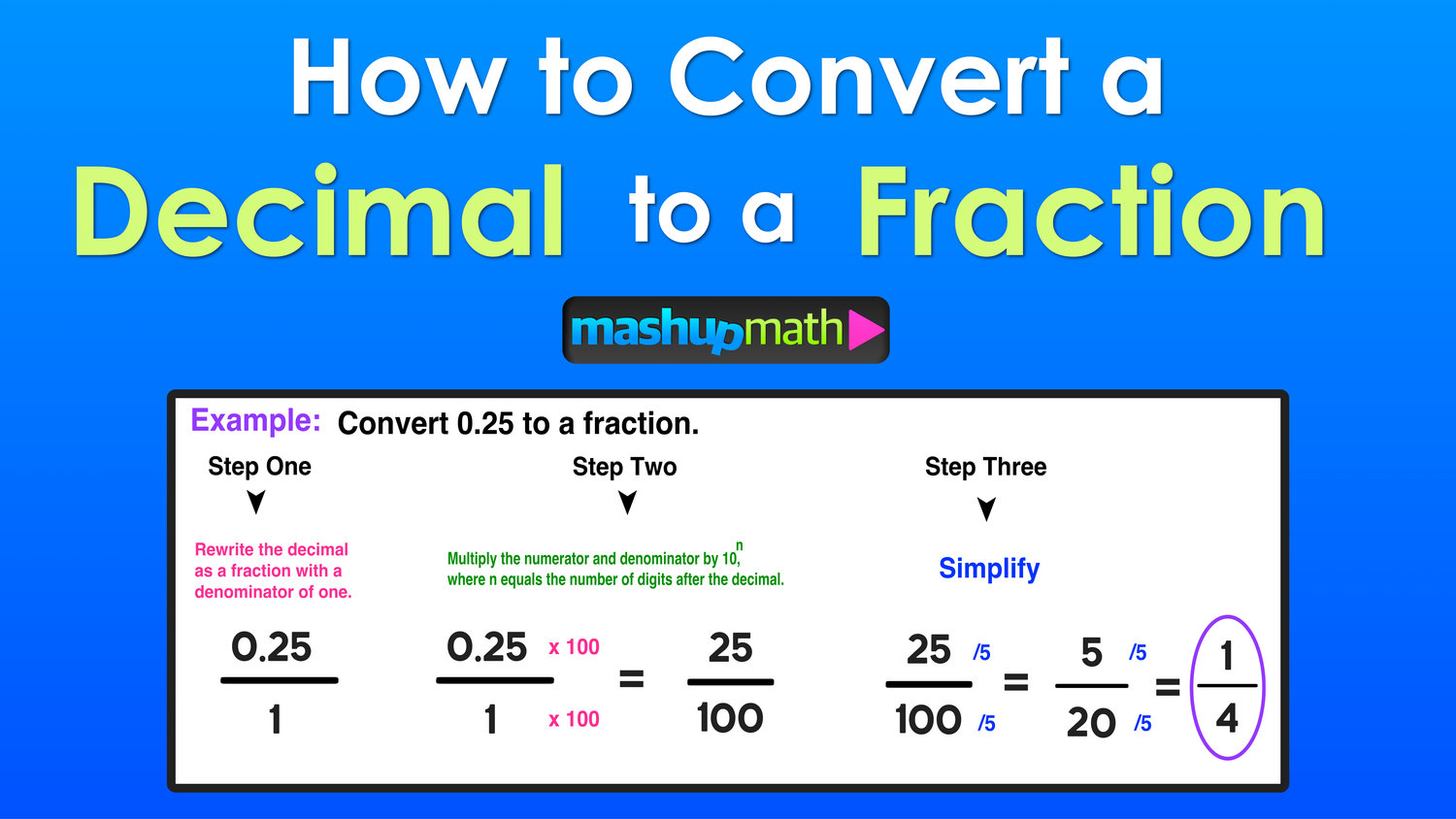
How do you convert a decimal to a fraction? Any decimal, even complicated-looking ones, can be converted to a fraction; you just need to follow a few steps. Below we explain how to convert both terminating decimals and repeating decimals to fractions.
Table of Contents
Converting a Terminating Decimal to a Fraction
A terminating decimal is any decimal that has a finite other of digits. In other words, it has an end. Examples include .5, .234, .864721, etc. Terminating decimals are the most common decimals you’ll see and, fortunately, they are also the easiest to convert to fractions.
Step 1
Write the decimal divided by one.
For example, say you’re given the decimal .55. Your first step is to write out the decimal so it looks like .55/1
Step 2
Next, you want to multiply both the top and bottom of your new fraction by 10 for every digit to the left of the decimal point.
In our example, .55 has two digits after the decimal point, so we’ll want to multiply the entire fraction by 10 x 10, or 100. Multiplying the fraction by 100/100 gives us 55/100.
Step 3
The final step is reducing the fraction to its simplest form. The simplest form of the fraction is when the top and bottom of the fraction are the smallest whole numbers they can be. For example, the fraction 3/9 isn’t in its simplest form because it can still be reduced down to ⅓ by dividing both the top and bottom of the fraction by 3.
The fraction 55/100 can be reduced by dividing both the top and bottom of the fraction by 5, giving us 11/20. 11 is a prime number and can’t be divided any more, so we know this is the fraction in its simplest form.
The decimal .55 is equal to the fraction 11/20.
Example
Convert .108 to a fraction.
After putting the decimal over 1, we end up with .108/1
Since .108 has three digits after the decimal place, we need to multiply the entire fraction by 10 x 10 x 10, or 1000. This gives us 108/1000.
Now we need to simplify. Since 108 and 1000 are both even numbers, we know we can divide both by 2. This gives us 54/500. These are still even numbers, so we can divide by 2 again to get 27/250. 27 isn’t a factor of 250, so the fraction can’t be reduced any more.
The final answer is 27/250.
Converting a Repeating Decimal to a Fraction
A repeating decimal is one that has no end. Since you can’t keep writing or typing the decimal out forever, they are often written as a string of digits rounded off (.666666667) or with a bar above the repeating digit(s) (.6).
For our example, we’ll convert .6667 to a fraction.
The decimal .6667 is equal to (.6), .666666667, .667, etc. They’re all just different ways to show that the decimal is actually a string of 6’s that goes on forever.
Step 1
Let x equal the repeating decimal you’re trying to convert, and identify the repeating digit(s).
So x=.6667
6 is the repeating digit, and the end of the decimal has been rounded up.
Step 2
Multiply by whatever value of 10 you need to get the repeating digit(s) on the left side of the decimal.
For .6667, we know that 6 is the repeating digit. We want that six on the left side of the decimal, which means moving the decimal place over one spot. So we multiply both sides of the equation by (10 x 1) or 10.
10x = 6.667
Note: You only want one “set” of repeating digit(s) on the left side of the decimal. In this example, with 6 as the repeating digit, you only want one 6 on the left of the decimal. If the decimal was 0.58585858, you’d only want one set of “58” on the left side. If it helps, you can picture all repeating decimals with the infinity bar over them, so .6667 would be (.6).
Step 3
Next we want to get an equation where the repeating digit is just to the right of the decimal.
Looking at x = .6667, we can see that the repeating digit (6) is already just to the right of the decimal, so we don’t need to do any multiplication. We’ll keep this equation as x = .6667
Step 4
Now we need to solve for x using our two equations, x = .667 and 10x = 6.667.
10x – x =6.667-.667
9x = 6
x = 6/9
x = 2/3
Example
Convert 1.0363636 to a fraction.
This question is a bit trickier, but we’ll be doing the same steps that we did above.
First, make the decimal equal to x, and determine the repeating digit(s). x = 1.0363636 and the repeating digits are 3 and 6.
Next, get the repeating digits on the left side of the decimal (again, you only want one set of repeating digits on the left). This involves moving the decimal three places to the right, so both sides need to be multiplied by (10 x 3) or 1000.
1000x = 1036.363636
Now get the repeating digits to the right of the decimal. Looking at the equation x = 1.0363636, you can see that there currently is a zero between the decimal and the repeating digits. The decimal needs to be moved over one space, so both sides need to be multiplied by 10 x 1.
10x = 10.363636
Now use the two equations, 1000x = 1036.363636 and 10x = 10.363636, to solve for x.
1000x – 10x = 1036.363636 – 10.363636
990x = 1026
x= 1026/990
Since the numerator is larger than the denominator, this is known as an irregular fraction. Sometimes you can leave the fraction as an irregular fraction, or you may be asked to convert it to a regular fraction. You can do this by subtracting 990/990 from the fraction and making it a 1 that’ll go next to the fraction.
1026/990 – 990/990 = 1(36/990)
x = 1(36/990)
36/990 can be simplified by dividing it by 18.
x = 1(2/55)
Conclusion
Decimals and fractions seem to be a world apart. They both have a fraction part and a whole part, but that doesn’t mean they’re equivalent. You can’t simply treat a decimal as a fraction. That’s where the decimals to fractions conversion would come in handy.