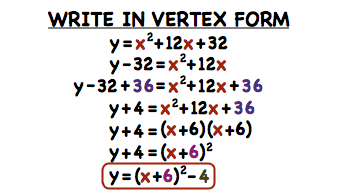
How to Convert Standard Form To Vertex Form – This article looks at the steps to convert standard form into vertex form by completing the square, using the quadratic formula. There are other methods to do this which you can learn about in this article.
In this mini-lesson, we will explore the process of converting standard form to vertex form and vice-versa.
The standard form of a parabola is y=ax2+bx+cy=ax2+bx+c.
The vertex form of a parabola is y=a(x−h)2+ky=a(x−h)2+k.
Here, the vertex form has a square in it.
So to convert the standard to vertex form we need to complete the square.
Let’s learn about the following in detail:
- Standard form to vertex form
- Vertex form to standard form
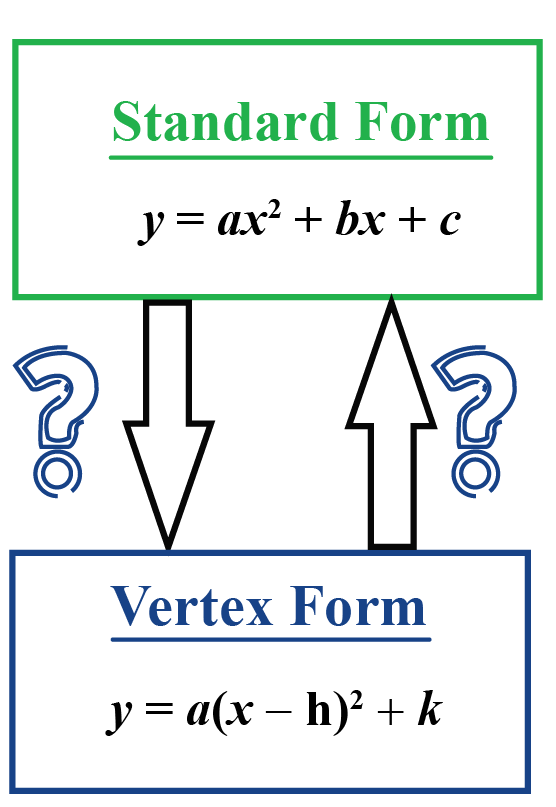
Table of Contents
How to Convert Standard Form To Vertex Form?
Standard Form
The standard form of a parabola is:
| y=ax2+bx+cy=ax2+bx+c |
Here, a,b,a,b, and cc are real numbers (constants) where a≠0a≠0.
xx and yy are variables where (x,y)(x,y) represents a point on the parabola.
Vertex Form
The vertex form of a parabola is:
| y=a(x−h)2+ky=a(x−h)2+k |
Here, a,h,a,h, and kk are real numbers where a≠0a≠0.
xx and yy are variables where (x,y)(x,y) represents a point on the parabola. Important Notes
Important Notes
- In the vertex form, (h,k)(h,k) represents the vertex of the parabola where the parabola has either maximum/minimum value.
- If a>0a>0, the parabola has minimum value at (h,k)(h,k) and
if a<0a<0, the parabola has maximum value at (h,k)(h,k).
Standard to Vertex Form
In the vertex form, y=a(x−h)2+ky=a(x−h)2+k, there is a “whole square.”
So to convert the standard form to vertex form, we just need to complete the square.
Let us learn how to complete the square using an example.
Example
Convert the parabola from standard to vertex form:
y=−3×2−6x−9y=−3×2−6x−9
Solution:
First, we should make sure that the coefficient of x2x2 is 11
If the coefficient of x2x2 is NOT 11, we will place the number outside as a common factor.
We will get:
y=−3×2−6x−9=−3(x2+2x+3)y=−3×2−6x−9=−3(x2+2x+3)
Now, the coefficient of x2x2 is 11
Step 1: Identify the coefficient of xx.

Step 2: Make it half and square the resultant number.

Step 3: Add and subtract the above number after the xx term in the expression.

Step 4: Factorize the perfect square trinomial formed by the first 3 terms using the suitable identity
Here, we can use x2+2xy+y2=(x+y)2×2+2xy+y2=(x+y)2.
In this case,x2+2x+1=(x+1)2×2+2x+1=(x+1)2
The above expression from Step 3 becomes:

Step 5: Simplify the last two numbers and distribute the outside number.
Here, −1+3=2−1+3=2
Thus, the above expression becomes:

This is of the form a(x−h)2+ka(x−h)2+k, which is in the vertex form.
Here, the vertex is, (h,k)=(−1,−6)(h,k)=(−1,−6). Tips and Tricks
Tips and Tricks
If the above process seems difficult, then use the following steps:
- Compare the given equation with the standard form (y=ax2+bx+cy=ax2+bx+c) and get the values of a,b,a,b, and cc.
- Apply the following formulas to find the values the values of hh and kk and substitute it in the vertex form (y=a(x−h)2+ky=a(x−h)2+k):
h=−b2ak=−D4ah=−b2ak=−D4a
Here, DD is the discriminant where, D=b2−4acD=b2−4ac.
Standard Form to Vertex Form Calculator
Here is the “Standard Form to Vertex Form Calculator.”
You can enter the equation of the parabola in the standard form. This calculator shows you how to convert it into the vertex form with a step-by-step explanation.
How to Convert Vertex Form to Standard Form?
We know that the vertex form of parabola is y=a(x−h)2+ky=a(x−h)2+k.
To convert the vertex to standard form:
| Expand the square, (x−h)2(x−h)2.Distribute aa.Combine the like terms. |
Example
Let us convert the equation y=−3(x+1)2−6y=−3(x+1)2−6 from vertex to standard form using the above steps:
y=−3(x+1)2−6y=−3(x+1)(x+1)−6y=−3(x2+2x+1)−6y=−3×2−6x−3−6y=−3×2−6x−9y=−3(x+1)2−6y=−3(x+1)(x+1)−6y=−3(x2+2x+1)−6y=−3×2−6x−3−6y=−3×2−6x−9
Solved Examples
| Example 1 |
Can we help Sophia to find the vertex of the parabola y=2×2+7x+6y=2×2+7x+6 by completing the square?
Solution
The given equation of parabola is y=2×2+7x+6y=2×2+7x+6.
To complete the square, first, we will make the coefficient of x2x2 as 11
We will take the coefficient of x2x2 (which is 22) as a common factor.
2×2+7x+6=2(x2+72x+3)→(1)2×2+7x+6=2(x2+72x+3)→(1)
The coefficient of xx is 7272
Half of it is 7474
Its square is (74)2=4916(74)2=4916
This term can also be found using (−b2a)2=(−72(2))2=4916(−b2a)2=(−72(2))2=4916
Add and subtract it after the xx term in (1):
2×2+7x+6=2(x2+72x+494−494+3)2×2+7x+6=2(x2+72x+494−494+3)
Factorize the trinomial made by the first three terms:
2×2+7x+3=2(x2+72x+4916−4916+3)=2((x+74)2−4916+3)=2((x+74)2−116)=2(x+74)2−182×2+7x+3=2(x2+72x+4916−4916+3)=2((x+74)2−4916+3)=2((x+74)2−116)=2(x+74)2−18
By comparing the final equation with the vertex form, a(x−h)2+ka(x−h)2+k:h=−74k=−18h=−74k=−18
Thus the vertex of the given parabola is:
| (h,k)=(−74,−18)(h,k)=(−74,−18) |
| Example 2 |
Though we helped Sophia to find the vertex of y=2×2+7x+6y=2×2+7x+6 in the above example, she is still not comfortable with this method.
Can we help her to find its vertex without completing the square?
Solution
The given equation of parabola is y=2×2+7x+6y=2×2+7x+6.
We will use the trick mentioned in the Tips and Tricks section of this page to find the vertex without completing the square.
Compare the given equation with y=2×2+7x+6y=2×2+7x+6:
a=2b=7c=6a=2b=7c=6
The discriminant is:D=b2−4ac=72−4(2)(6)=1D=b2−4ac=72−4(2)(6)=1
We will find the coordinates of the vertex using the formulas:
h=−b2a=−72(2)=−74k=−D4a=−14(2)=−18h=−b2a=−72(2)=−74k=−D4a=−14(2)=−18
Therefore, the vertex of the given parabola is:
| (h,k)=(−74,−18)(h,k)=(−74,−18) |
Note that the answer is same as that of Example 1.
| Example 3 |
Find the equation of the following parabola in the standard form:

Solution
We can see that the parabola has the maximum value at the point (2,2)(2,2).
So the vertex of the parabola is,(h,k)=(2,2)(h,k)=(2,2)
So the vertex form of the above parabola is,y=a(x−2)2+2→(1)y=a(x−2)2+2→(1)
To find aa here, we have to substitute any known point of the parabola in this equation.
The graph clearly passes through the point (x,y)=(1,0)(x,y)=(1,0).
Substitute it in (1):
0=a(1−2)2+20=a+2a=−20=a(1−2)2+20=a+2a=−2
Substtute it back into (1) and expand the square to convert it into the standard form:
y=−2(x−2)2+2y=−2(x−2)(x−2)+2y=−2(x2−4x+4)+2y=−2×2+8x−8+2y=−2×2+8x−6y=−2(x−2)2+2y=−2(x−2)(x−2)+2y=−2(x2−4x+4)+2y=−2×2+8x−8+2y=−2×2+8x−6
Thus, the standard form of the given parabola is:
| y=−2×2+8x−6y=−2×2+8x−6 |
Conclusion
When completing the square, you can avoid using algebra by using standard form, completing the square on the standard form, and then switching to vertex form. This article shows how to convert standard form to vertex form.