Not everything is so easy to read when it is written down in numbers. How long were you supposed to spend on that math problem? Or how many gallons of water are in the sea? If you want to know something about how to convert how to convert scientific notation, then check out How To Convert Scientific Notation
When numbers are too long and unwieldy to be easily expressed as a decimal, they can be simplified using scientific notation. Learn how to convert numbers to scientific notation. Review several examples to understand the process and benefits of converting both large and small numbers using scientific notation. Updated: 10/30/2021
We are going to take a look at scientific notation. This is a special way to write numbers. Who uses scientific notation? If you guessed scientists, you are 100% correct! Scientists use it because it is a way to write out really large and really small numbers without having to write a whole bunch of zeroes.
What does it look like? You will know that you are looking at scientific notation when you see a number multiplied by 10 to a power. For example, 2.7 * 10^3 is the number 2,700 written in scientific notation. See the multiplication by 10 to a power? We’ll see later how we get the number of the power. Another example is 2.7 * 10^-3, which is the number 0.0027 written in scientific notation.
While it’s easy for us to write and read 2,700 and 0.0027, when we get to much larger numbers, scientific notation makes it much easier to write them. For example, instead of writing 1,988,000,000,000,000,000,000,000,000,000, we could just write 1.988 * 10^30. Doesn’t that look much neater? This really large number is actually the mass of the sun in kilograms.
Scientific notation is a technique of expressing gigantic or incredibly small numbers in a simpler form. Although scientific notation is one of the oldest mathematical operation, nowadays, it is used by engineers, scientists and mathematicians to ease the computation.
The general form of writing a number in scientific notation is: a × 10n where 1 ≤ a ≤ 10 and n is any integer. The number a which known as coefficient must be greater than 1 and less than 10.
An example of scientific notation is 2.5 x 106 which is equivalent to expressing the number as 2500000. In this example, 2.5 is the coefficient and the first digit of the coefficient is known as the mantissa. The number 2 is the mantissa in 2.5 x 106.
Table of Contents
How to Convert to Scientific Notation?
In this article, we shall learn the rules and procedures of converting numbers to scientific notation and therefore, you will be able to convert any number you come across on your own. Conversion of numbers to scientific notation is quite simple and easy.
Let us take a look into these steps for converting numbers to scientific notation:
Latest Videos
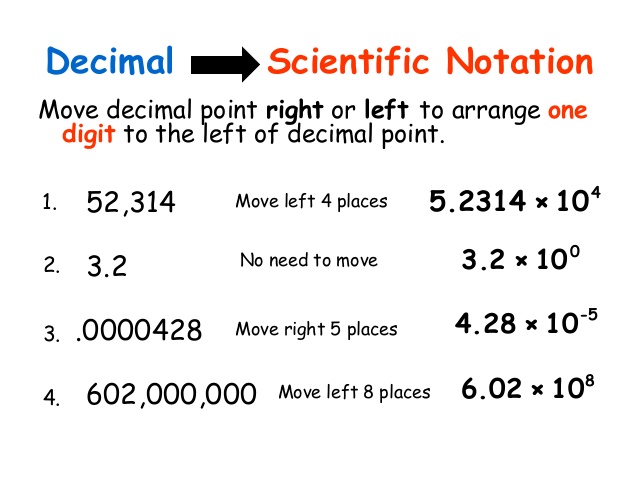
- To convert a number to scientific notation, place or move the decimal point of a number until the coefficient of the number is greater than 1 and less than 10.
- Record down the coefficient (a) and count the number of steps the decimal point was moved.
- The number of steps moved (n) is taken as the exponent.
- Moving the decimal point to the right gives a negative exponent, whereas moving the decimal point to the left makes a positive exponent.
Example 1
Consider a big number 3,400,000. To convert this number into scientific notation:
- Place a decimal by counting the steps to the left until the coefficient of the number is between 1 and 9.
- Count the number of steps moved. This will be the power of the base 10.
- In this case, the coefficient is 3.4 and the 6 steps are moved.
- Multiply the coefficient by 106,
- Therefore, the answer is 3. 4 x 10 6
Example 2
Consider another scenario of a small number 0. 00041.In this case the scientific notation is negative because the number is less than 1.
- Move the decimal point to the right until you find the coefficient to be greater than 1 and less than 10.
- The coefficient is therefore, 4.1 and the steps moved are 4. This implies that, the power of the base 10 will be 4.
- Multiply the coefficient with the base: 4.1 x 10-4. The negative exponent indicates that, we moved to the right,
- Thus, the answer is 4.1 x 10-4
Example 3
Convert the following number to scientific notation:
81 900 000 000 000
- Place the decimal to the number by counting from the right to the left.
- The coefficient of the number should be between 1 and 10, and in this case 8. 19 is our coefficient
- Count the number of steps moved because this will be the power of the base 10.
- Multiply the coefficient by the base: 8.19 x 10 13
- So, the answer is 8.19 x 10 13
Another Way Of Converting to Scientific Notation
It’s quite easy to convert our regular numbers into scientific notation. Let’s convert the number 3,400,000 into scientific notation. First, we write out the first few non-zero digits and place a decimal after the first digit. We have 3.4. Now, we’re going to count how many digits there are after the 3. We have 6. This tells us that our power is 6. So, we finish off by writing 3.4 * 10^6. This tells us that we have a total of 6 digits after the 3. We would add zeroes until we have a total of 6 digits after the decimal.
If our number is smaller than 1, our scientific notation will have a negative exponent. Let’s convert the number 0.00041 into scientific notation. This time, instead of taking the first few non-zero digits, we are now taking the last few non-zero digits and placing a decimal after the first non-zero digit. We have 4.1. Now, we count the number of digits away from our beginning decimal. We get 4. So, this tells us that our power is -4. Our scientific notation, then, is 4.1 * 10^-4.
Another way you can remember whether the power is positive or negative is by the direction you need to move the decimal point. If you need to move it to the left, then your power is positive. If you need to move it to the right, then your power is negative.
Conclusion
How to convert scientific notation to standard notation can be challenging, but you can do it if you remember what you are doing. It is important to write these problems in the correct number form. Scientific notation is just a short way of writing very large or very small numbers.