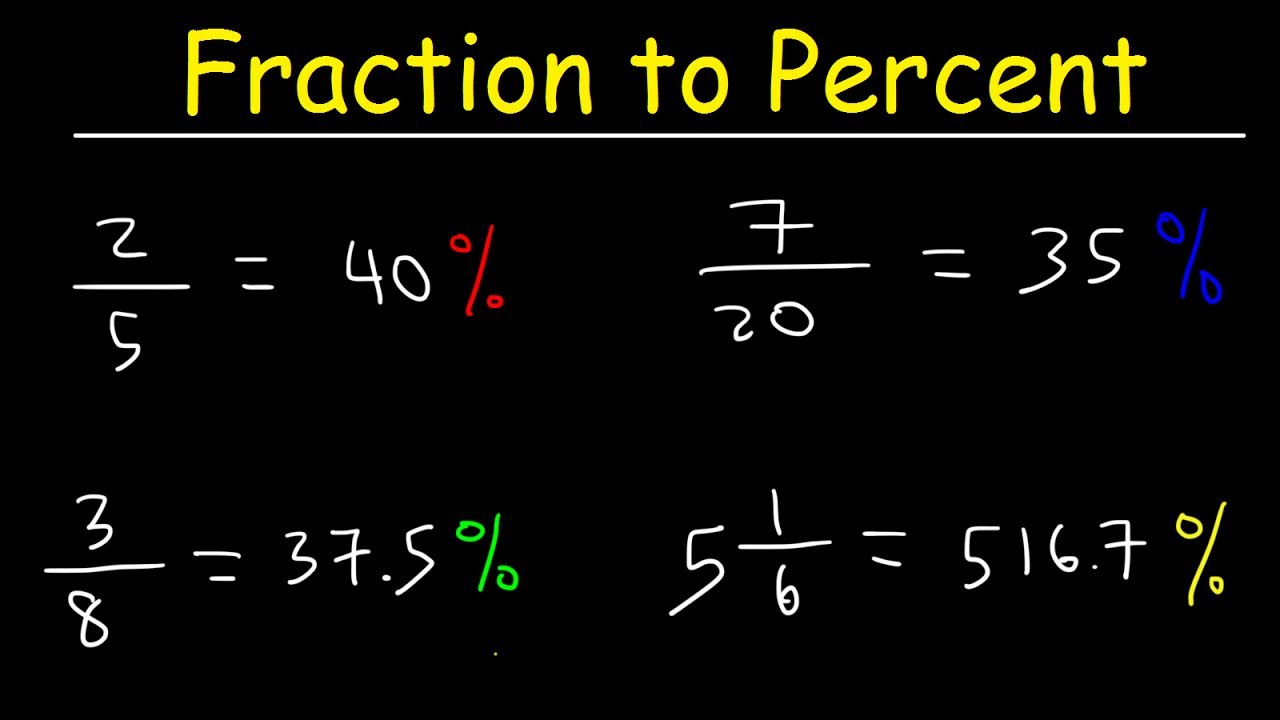
We need to learn “How To Convert a Fraction To a Percent”. In this article, you will get an explanation on “how to convert a mixed fraction to a percent” and “how to convert a proper fraction to a percent”.
Have you got fractions and don’t know how to convert them to percentages? Like, look at the fraction 199/600. You want to know how to convert fraction to percentage, so you could do further math with this fraction. Chances are – you have a lot of problems with converting fractions into a percentage. Learn in this post how to convert fraction into percentage.
Table of Contents
What is a Percentage?
The term ‘per cent’, or the Latin word ‘percentage’, means ‘out of a hundred’. In mathematics, percentages are used like fractions and decimals, as ways to describe parts of a whole. You can therefore consider each ‘whole’ as broken up into 100 equal parts, each one of which is a single percent. The symbol % is used to show that a number is a percentage, and less commonly the abbreviation ‘pct’ may be used.
We use percentages to make calculations easier. It is much simpler to work with parts of 100 than thirds, twelfths and so on, especially because quite a lot of fractions do not have an exact (non-recurring) decimal equivalent. Importantly, this also makes it much easier to make comparisons between percentages (which all effectively have the common denominator of 100) than it is between fractions with different denominators. This is partly why so many countries use a metric system of measurement and decimal currency.
What is a Fraction?
A fraction has two parts. The number on the top of the line is called the numerator. It tells how many equal parts of the whole or collection are taken. The number below the line is called the denominator. It shows the total divisible number of equal parts the whole into or the total number of equal parts which are there in a collection.
There are different types of fractions. Fractions with numerator 1 are called unit fractions. Fractions in which the numerator is less than the denominator are called proper fractions. Fractions in which the numerator is more than or equal to the denominator are called improper fractions. Mixed fractions consist of a whole number along with a proper fraction.
How to Convert a Fraction to Percentage
The Simplest Method is to Use a Calculator
| | Steps: Divide the top of the fraction by the bottom,Then multiply the result by 100 and read off the answer ! |
Example: What is 58 as a percent?
Get your calculator and type in “5 ÷ 8 =”, the calculator should show 0.625
Then multiply by 100 and your answer is: 62.5%
(Remember to put the “%” sign so people know it is “per 100”)
OR you can multiply the top by 100 first then divide by the bottom number:
Example: What is 5/8 as a percent?
Multiply 5 by 100 first, then divide by 8
500 ÷ 8 = 62.5%
(Remember that “%” sign!)
Or Move the Decimal 2 Places
After dividing, instead of multiplying by 100 we can just move the decimal point 2 places to the right, then add the %
Example: Convert 1/8 to a percentage
Divide 1 by 8:
1 ÷ 8 = 0.125
Move the decimal point 2 places to the right

Remember to add the “%” sign: 12.5%
Another Method
Percent means “per 100”, so try to change the fraction to ?/100 form.
Follow these steps:
| Step 1: Find a number you can multiply the bottom of the fraction by to get 100. |
| Step 2: Multiply both top and bottom of the fraction by that number. |
| Step 3. Then write down just the top number with the “%” sign. |
Example 1: Convert 3/4 to a Percent
Step 1: We can multiply 4 by 25 to become 100
(why 25? because 100 divided by 4 is 25)
Step 2: Multiply top and bottom by 25:
Step 3: Write down 75 with the percent sign:
3/4*25/25 = 75/100
Answer = 75%
Example 2: Convert 3/16 to a Percent
Step 1: We have to multiply 16 by 6.25 to become 100
(why 6.25? because 100 divided by 16 is 6.25)
Step 2: Multiply top and bottom by 6.25:
3/16*6.25/6.25 = 18.75/100
Step 3: Write down 18.75 with the percentage sign:
Answer = 18.75%
Another Method: Proportions
Because a percent is actually a ratio (parts per 100) we can also use Proportions to do the conversion.
First, put what you know into this form:
Top of Fraction/Bottom of Fraction = Percent/100
Then solve using “multiply across the known corners, divide by the third number”:
Example: Convert 3/16 to percent
Fill in what you know:
3/16 = Percent/100
Multiply across the known corners, then divide by the third number. The “known corners” are top left and bottom right:
Percent = (3 × 100) / 16
= 300 / 16
= 18.75%
Answer: 3/16 is 18.75% (same answer as the previous example!)
Another Method
- Use division to convert the fraction to a decimal:
1/4 = 1 ÷ 4 = 0.25 - Multiply by 100 to get percent value:
0.25 × 100 = 25%
1. Convert the fraction to a decimal number
The fraction bar between the top number (numerator) and the bottom number (denominator) means “divided by.” So converting a fraction such as 1/4 to a decimal means you need to solve the math: 1 divided by 4.
1 ÷ 4 = 0.25
2. Multiply by 100 to convert decimal number to percent
0.25 × 100 = 25%
You can reduce a fraction before converting to a decimal but it’s not necessary because the answer will be the same. If you need to do the conversion by long division, reducing might make the math easier.
For example, 6/12 = 6 ÷ 12 = 0.50. If you solve this with a calculator then it is easy to get the answer. However, if you solve this by hand or in your head reducing 6/12 = 1/2 may make the problem easier and you may even recognize that 1/2 = 0.50.
Multiplying 0.50 by 100 means that 6/12 = 50%.
Another Method
- Multiply the fraction by 100
- Divide the numerator and denominator by the denominator
For example:
(i) Express 3/4 as a percent.
Solution:
¾ × 100
= (3 × 100)/4
= 300/4
= 300/4 ÷ 4/4
[Divide the numerator and denominator by 4]
= 75%.
Answer: 75%
(ii) Express 12/5 as a percent.
Solution:
12/5 × 100
= (12 × 100)/5
= 1200/5
= 1200/5 ÷ 5/5
[Divide the numerator and denominator by 5]
= 240%.
Answer: 240%
(iii) Express 19/4 as a percent.
Solution:
19/4 × 100
= (19 × 100)/4 = 1900/4
= 1900/4 ÷ 4/4
[Divide the numerator and denominator by 4]
= 475%.
Answer: 475%
Conclusion
Fractions are a part of almost everyone’s lives. In math, we see them all the time. As kids, we learn basic fractions… Learning how to convert fraction to percentage without a calculator is a basic knowledge you need to know if you want to become a professional in mathematics and engineering.Open document settingsOpen publish panel