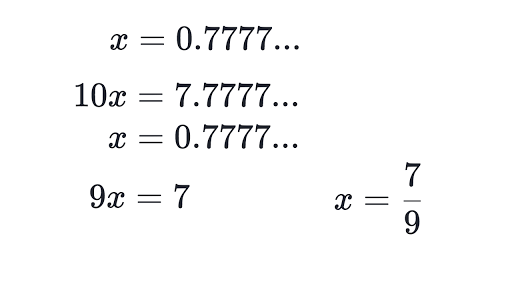Did you know that a full 90% of students who take a class on converting repeating decimals to fractions don’t even know how to convert repeating decimals into fractions? This doesn’t mean that the rest of 10% is limited to this subject, though. In fact, these students are achieving with flying colors at their current classrooms. Thankfully, this blog post has provided some great knowledge about how to convert repeating decimals into fractions.
A repeating decimal, also known as a recurring decimal, is a decimal number that has a digit or digits that infinitely repeat at regular intervals. Repeating decimals can be tricky to work with, but they can also be converted into a fraction. Sometimes, repeating decimals are indicated by a line over the digits that repeat. The number 3.7777 with 7 repeating, for instance, can also be written as 3.7. To convert a number like this to a fraction you write it as an equation, multiply, subtract to remove the repeating decimal, and solve the equation.
Table of Contents
Part 1 Converting Basic Repeating Decimals
- 1 Locate the repeating decimal. For instance, the number 0.4444 has a repeating decimal of 4. It is a basic repeating decimal in the sense that there’s no non-repeating portion to the decimal number. Count how many repeating digits there are in the pattern.
- Once your equation is written, you will multiply it by 10^y, where y equals the number of repeating digits in the pattern.[1]
- In the example of 0.4444, there is one digit that repeats, so you will multiply the equation by 10^1.
- For a repeating decimal of 0.4545, there are two digits that repeat, and you would, therefore, multiply your equation by 10^2.
- For three repeating digits, multiply by 10^3, etc.
- 2 Rewrite the decimal as an equation. Write it out so that x equals the original number. [2] In this instance, the equation is x = 0.4444. Since there’s only one digit in the repeating decimal, multiply the equation by 10^1 (which equals 10).[3]
- In the example where x = 0.4444, then 10x = 4.4444.
- With the example x = 0.4545, there are two repeating digits, so you multiply both sides of the equation by 10^2 (which equals 100), giving you 100x = 45.4545.
- 3 Remove the repeating decimal. You accomplish this by subtracting x from 10x. Remember that whatever you do to one side of the equation must be done to the other, so:[4]
- 10x – 1x = 4.4444 – 0.4444
- On the left side, you have10x – 1x = 9x. On the right side, you have 4.4444 – 0.4444 = 4
- Therefore, 9x = 4
- 4 Solve for x. Once you know what 9x equals, you can determine what x equals by dividing both sides of the equation by 9:
- On the left side of the equation you have 9x ÷ 9 = x. On the right side of the equation you have 4/9
- Therefore, x = 4/9, and the repeating decimal 0.4444 can be written as the fraction 4/9.
- 5 Reduce the fraction. Put the fraction in its simplest form (if applicable) by dividing both the numerator and denominator by the greatest common factor.[5]
- In the example of 4/9, that is the simplest form.
Part 2 Converting Numbers With Repeating and Non-Repeating Decimals
- 1 Determine the repeating digits. It’s not uncommon for a number to have non-repeating digits before the repeating decimal, but these can still be converted into fractions.
- For example, take the number 6.215151. Here, 6.2 is non-repeating, and the repeating digits are 15.
- Again take note of how many repeating digits there are in the pattern, because you will multiply by 10^y based on that number.
- In this example, there are two repeating digits, so you will multiply your equation by 10^2.
- 2 Write the problem as an equation and subtract the repeating decimals. Again, if x = 6.215151, then 100x = 621.5151. To remove the repeating decimals, subtract from both sides of the equation:
- 100x – x (= 99x) = 621.5151 – 6.215151 (= 615.3)
- Therefore, 99x = 615.3
- 3 Solve for x. Since 99x = 615.3, divide both sides of the equation by 99. This gives you x = 615.3/99.
- 4 Remove the decimal in the numerator. Do this by multiplying the numerator and denominator by 10^z, where z equals the number of decimal places you must move to eliminate the decimal. In 615.3, you have to move the decimal by one place, meaning you multiply the numerator and denominator by 10^1:
- 615.3 x 10 / 99 x 10 = 6153/990
- Reduce the fraction by dividing the numerator and denominator by the highest common factor, which in this case is 3, giving you x = 2,051/ 330
Another method of onverting repeating decimals to fractions
When converting repeating decimals to fractions, just follow the five steps below carefully.
Step 1:
Let x equal the repeating decimal you are trying to convert to a fraction.
Step 2:
Examine the repeating decimal to find the repeating digit(s).
Step 3:
Place the repeating digit(s) to the left of the decimal point.
Step 4:
Place the repeating digit(s) to the right of the decimal point.
Step 5:
Using the two equations you found in step 3 and step 4, subtract the left sides of the two equations. Then, subtract the right sides of the two equations
As you subtract, just make sure that the difference is positive for both sides.
Now let’s practice converting repeating decimals to fractions with two good examples.
Example #1:
What rational number or fraction is equal to 0.55555555555
Step 1:
x = 0.5555555555
Step 2:
After examination, the repeating digit is 5.
Step 3:
To place the repeating digit ( 5 ) to the left of the decimal point, you need to move the decimal point 1 place to the right.

https://f73477131963652b68405ca45691938a.safeframe.googlesyndication.com/safeframe/1-0-38/html/container.html
Technically, moving a decimal point one place to the right is done by multiplying the decimal number by 10.
When you multiply one side by a number, you have to multiply the other side by the same number to keep the equation balanced.
Thus, 10x = 5.555555555
Step 4:
Place the repeating digit(s) to the right of the decimal point.
Look at the equation in step 1 again. In this example, the repeating digit is already to the right, so there is nothing else to do.
x = 0.5555555555
Step 5:
Your two equations are:
10x = 5.555555555
x = 0.5555555555
10x – x = 5.555555555 − 0.555555555555
9x = 5
Divide both sides by 9.
x = 5/9
Example #2:
What rational number or fraction is equal to 1.04242424242
Step 1:
x = 1.04242424242
Step 2:
After examination, the repeating digit is 42.
Step 3:
To place the repeating digit ( 42 ) to the left of the decimal point, you need to move the decimal point 3 places to the right.
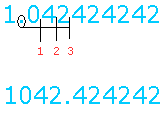
Again, moving a decimal point three places to the right is done by multiplying the decimal number by 1000.
When you multiply one side by a number, you have to multiply the other side by the same number to keep the equation balanced
Thus, 1000x = 1042.42424242
Step 4:
Place the repeating digits to the right of the decimal point.
In this example, the repeating digit is not immediately to the right of the decimal point.
Look at the equation in step 1 one more time and you will see that there is a zero between the repeating digit and the decimal point.
To accomplish this, you have to move the decimal point 1 place to the right.
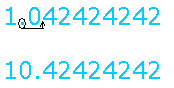
This is done by multiplying both sides by 10.
10x = 10.4242424242
Step 5:
Your two equations are:
1000x = 1042.42424242
10x = 10.42424242
1000x – 10x = 1042.42424242 − 10.42424242
990x = 1032
Divide both sides by 990
x = 1032/990
To master this lesson about converting repeating decimals to fractions, you will need to study the two examples above carefully and practice with other examples.
Common pitfalls to avoid when converting repeating decimals to fractions
- Forgetting to put the decimal point right before the repeating digit(s).
- When subtracting the two equations, forgetting to subtract the smaller one from the bigger one.
- Not keeping good track of the number of places the decimal point was moved.
- Thinking that the repeating digit(s) is 8 if the decimal is 0.08
Conclusion
Have you ever had to convert repeating decimals to fractions but didn’t know where to look? Have you tried various ways for converting repeating decimals to fractions, only to get frustrated and think it’s impossible? Well, it’s not. With the right method, you can easily convert repeating decimals into fractions in just a few minutes. Cool!