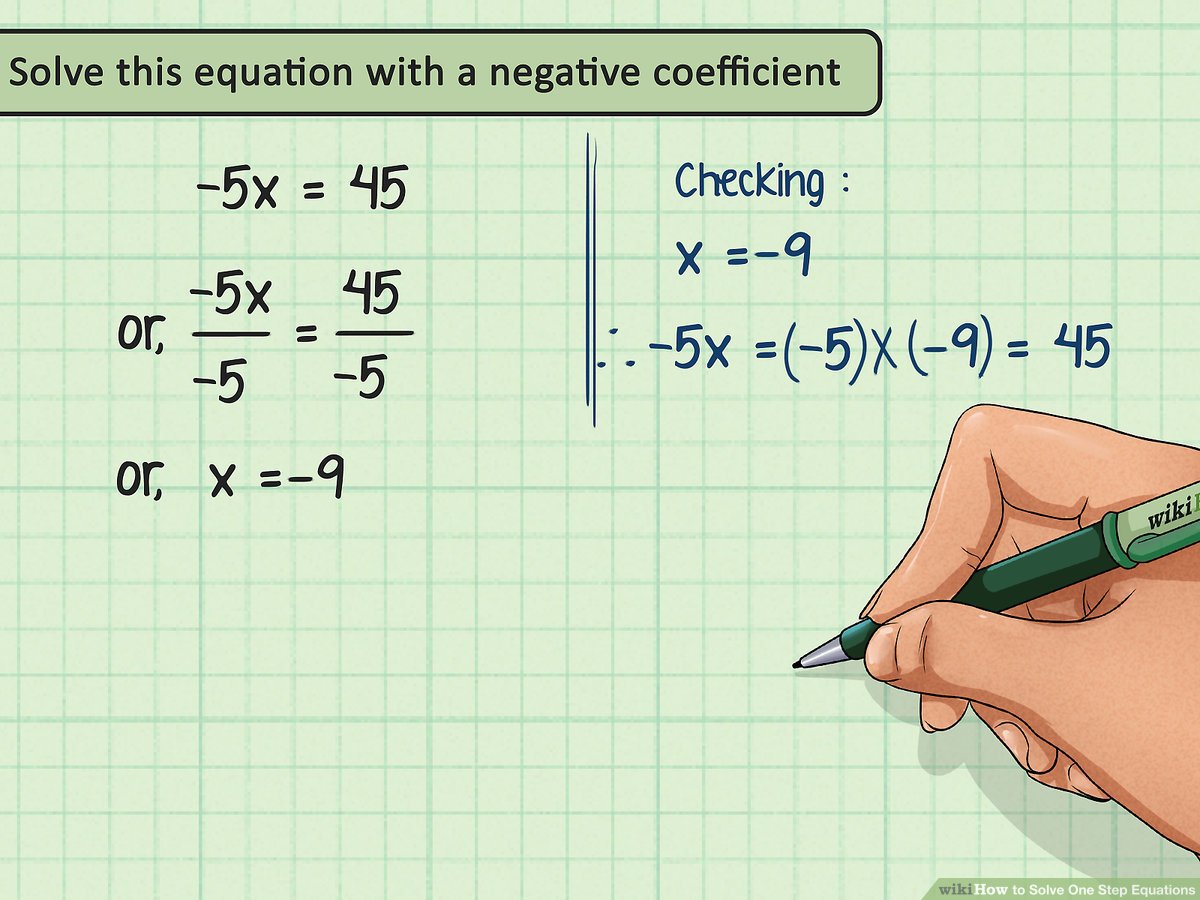How to Solve One-Step Equations With Fractions – When it comes to solving one step equations, the number of steps we take is exactly the number that the name implies – one! One step equations are equations that involve adding, subtracting, multiplying, or dividing both sides of the equation by the same number, variable, or term in order to isolate and solve for the variable.
A one-step equation is an equation that only requires one step to solve! You can solve a one-step equation with addition, subtraction, multiplication, or division.
Table of Contents
Examples of one-step equations
Below are four simple examples of one-step equations:
| x+3=15 x + 3 = 15 x+3=15 | 6+y=10 6 + y = 10 6+y=10 |
| 5z=35 5z = 35 5z=35 | x7=3 \dfrac{x}{7} = 3 7x=3 |
Notice how each of the four examples above has only one operation on the left side of the equation sign.Free Practice with One-Step Equations on Albert

What does it mean to “solve” an equation?
In order to solve an equation, one must find a value of the variable that makes an equation true, or a solution. To find a solution for a one-step equation, you will use inverse operations.
Examples of inverse operations are:
Addition↔Subtraction \text{Addition} \leftrightarrow \text{Subtraction} Addition↔Subtraction
Multiplication↔Division \text{Multiplication} \leftrightarrow \text{Division} Multiplication↔Division
When working with inverse operations, it is important to remember that whatever you do to one side of the equation, you must also do to the other.
Solving one-step equations (basics)
Solve one-step equation with addition
Solve for x x x in the following equation:
x+8=14 x + 8 = 14 x+8=14
To find the solution for this equation, we must first get x x x by itself on the left side. Since x x x has 8 8 8 added to it, we must use the inverse operation of addition, subtraction. So we will subtract 8 8 8 from each side.
x+8=14 x + 8 = 14 x+8=14
x+8−8 =14−8 x + 8 \mathbf{\color{red}{- 8}} = 14 \mathbf{\color{red}{- 8}} x+8−8 =14−8
x=6 x = 6 x=6
Original equation
Subtract 8 8 8 from both sides
Simplify
To check your answer, you can simply substitute 6 6 6 into the variable to see if the equation is true:
x+8=14 x + 8 = 14 x+8=14
(6)+8=14 (6) + 8 = 14 (6)+8=14
14=14✓ 14 = 14 \checkmark 14=14✓
Thus, x=6 x = 6 x=6 is the correct solution.
Solve one-step equation with subtraction
Solve for y y y in for the following equation:
y−12=7 y – 12 = 7 y−12=7
Again, we will use the inverse operation to get y y y by itself and solve the equation. Remember, the inverse operation of subtraction is addition. Therefore to solve, we will add 12 12 12 to both sides.
y−12=7 y – 12 = 7 y−12=7
y−12+12 =7+12 y – 12 \mathbf{\color{red}{ + 12}} = 7 \mathbf{\color{red}{+ 12}} y−12+12 =7+12
y=19 y = 19 y=19
Original equation
Add 12 12 12 to both sides
Simplify
To check your solution, simply substitute 19 19 19 into y y y:
y−12=7 y – 12 = 7 y−12=7
(19)−12=7 (19) – 12 = 7 (19)−12=7
7=7✓ 7 = 7 \checkmark 7=7✓
Therefore, y=19 y = 19 y=19 is the correct solution.Free Practice with One-Step Equations (Add or Subtract) on Albert
Solve one-step equation with multiplication
Solve for m m m in the following equation:
4m=20 4m = 20 4m=20
Since 4m 4m 4m implies “Four times m m m”, we will have to use the inverse operation of multiplication, which is division. Therefore to solve, we will simply divide each side by 4 4 4.
4m=20 4m = 20 4m=20
4m4=204 \dfrac{4m}{\color{red}{4}} = \dfrac{20}{\color{red}{4}} 44m=420
m=5 m = 5 m=5
Original equation
Divide each side by 4 4 4
Simplify
To check you answer, simply substitute 5 5 5 into m m m.
4m=20 4m = 20 4m=20
4(5)=20 4 (5) = 20 4(5)=20
20=20✓ 20 = 20 \checkmark 20=20✓
Therefore, m=5 m = 5 m=5 is the correct solution.
Solve one-step equation with division
Solve for z z z in the following equation:
z3=10 \dfrac{z}{3} = 10 3z=10
Since z3 \dfrac{z}{3} 3z implies “z z z divided by three”, we will use the inverse operation of division, multiplication. Therefore, to solve for z z z, we will multiply each side by 3 3 3.
z3=10 \dfrac{z}{3} = 10 3z=10
z3⋅3 \dfrac{z}{3} \cdot \color{red}{3}3z⋅3 =10⋅3= 10 \cdot \color{red}{3} =10⋅3
z=30 z = 30 z=30
Original equation
Multiply each side by 3 3 3
Simplify
To check you answer, simply substitute 30 30 30 into z z z:
z3=10 \dfrac{z}{3} = 10 3z=10
(30)3=10 \dfrac{(30)}{3} = 10 3(30)=10
10=10✓ 10 = 10 \checkmark 10=10✓
Therefore, z=30 z = 30 z=30 is the correct solution.Free Practice with One-Step Equations (Multiply or Divide) on Albert
For some more examples, check out this YouTube video from “Math with Mr. J”: https://www.youtube.com/embed/L0_K89UJfJY?feature=oembed
Solving one-step equations with fractions
When a one-step equation involves fractions, there are two ways that we can solve the equation. The first method treats the fraction the same as our division example above. For instance, we will solve for x x x in the following equation:
15x=6 \dfrac{1}{5}x = 6 51x=6
Since we can see 15x \frac{1}{5}x 51x as the same as “x x x divided by five”, we will simply use the inverse operation of division, multiplication. We can multiply each side by 5 5 5.
5⋅15x=5⋅6 5 \cdot \dfrac{1}{5}x = 5 \cdot 6 5⋅51x=5⋅6
x=30 x = 30 x=30
To check your answer, simply substitute 30 30 30 into x x x:
15⋅30=6 \dfrac{1}{5} \cdot 30 = 6 51⋅30=6
6=6✓ 6 = 6 \checkmark 6=6✓
Therefore, x=30 x = 30 x=30 is a correct solution. Free Practice with One-Step Equations on Albert
But, what if the fraction has a numerator that is not 1 1 1?
Great question We can still solve the equation in one step! When solving an equation, our goal is to isolate the variable (meaning get the variable by itself). This implies that we want the coefficient in front of the variable to be 1 1 1. In order to do this in one step, we multiply both sides of the equation by the reciprocal of the fraction. (A reciprocal of a fraction flips the numerator and denominator of a fraction.)
Let’s see an example: solve for t t t in the following equation:
23t=8 \dfrac{2}{3}t = 8 32t=8
As noted above, to solve for t t t we will multiply both sides of the equation by the reciprocal of 23 \dfrac{2}{3} 32, which is 32 \dfrac{3}{2} 23:
23t=8 \dfrac{2}{3}t = 8 32t=8
32⋅23t=32⋅8 \textcolor{red}{\dfrac{3}{2}} \cdot \dfrac{2}{3}t = \textcolor{red}{\dfrac{3}{2}} \cdot 8 23⋅32t=23⋅8
t=242 t = \dfrac{24}{2} t=224
t=12 t = 12 t=12
To check your answer, simply substitute 12 12 12 into t t ts
23⋅12=8 \dfrac{2}{3} \cdot 12 = 8 32⋅12=8
243=8 \dfrac{24}{3} = 8 324=8
8=8✓ 8 = 8 \checkmark 8=8✓
Therefore, t=12 t = 12 t=12 is the correct solution.
Solving one-step equations with integers
Sometimes, a one step equation will contain an integer value on one or both sides. (Remember, an integer can be positive, negative, or zero without any fractional part). In this situation, we can use the same techniques for solving one-step equations!
Solve for d d d in the following equation:
−8d=80 -8d = 80 −8d=80
Since an equation is not solved until the variable is by itself, if we simply divide by 8 8 8, we would still end up having −d -d−d on the left-hand side. Therefore, to solve this equation we must divide both sides by −8 -8 −8 as shown below
−8d−8=80−8 \dfrac{-8d}{-8} = \dfrac{80}{-8} −8−8d=−880
d=−10 d = -10 d=−10
To check your answer, simply substitute −10 -10 −10 into d d d:
−8⋅−10=80 -8 \cdot -10 = 80 −8⋅−10=80
80=80✓ 80 = 80 \checkmark 80=80✓
Therefore, d=−10 d = -10 d=−10 is the correct solution.
What about addition or subtraction problems with integer values? For example, solve for w w w in the following equation:
−5+w=10 -5 + w = 10 −5+w=10
Since our ultimate goal is to get w w w by itself, we need to eliminate the −5 -5 −5. To eliminate this integer value, we will add 5 5 5 to each side, as shown below:
−5+5+w=10+5 -5 \textcolor{red}{+ 5} + w = 10 \textcolor{red}{+ 5} −5+5+w=10+5
w=15 w = 15 w=15
To check your answer, simply substitute 15 15 15 in for w w w
−5+15=10 -5 + 15 = 10 −5+15=10
10=10✓ 10 = 10 \checkmark 10=10✓
Therefore, w=10 w = 10 w=10 is the correct solution.
Solving one-step equation word problems
We can model many real-life applications with a one-step equation. Once we have created an equation based on the information in the word problem, we simply solve the equation as we have above.
For example, model the following situation with an equation and find a solution that makes the situation true.
Word Problem Example 1
| Mark wanted to find out how much his cat weighed, but was struggling to get the cat to hold still on the scale. Therefore, he devised a plan to weigh himself and the cat together to figure out the cat’s weight. First, he weighs himself on the scale and finds his weight to be 150 lbs. 150 \text{ lbs.} 150 lbs. Then, he holds the cat and steps on the scale and finds the combined weight to be 165 lbs. 165 \text{ lbs.} 165 lbs.. Create an equation that models the situation and solve the equation to find, c c c, the cat’s weight. |
Solution: To model the following situation, we will create an equation to show the combined weights of Mark and his cat. We will represent the cat’s weight with the variable, c c c
c+150=165 c + 150 = 165 c+150=165
To solve for c c c, we will do the inverse operation of addition and subtract 150 150 150 from each side:
c+150−150=165−150 c + 150 \textcolor{red}{-150} = 165 \textcolor{red}{-150} c+150−150=165−150
c=15 lbs. c = 15 \text{ lbs.} c=15 lbs.
To check you answer, simply plug 15 15 15 into c c c:
15+150=165 15 + 150 = 165 15+150=165
165=165✓ 165 = 165 \checkmark 165=165✓
Therefore, c=15 lbs. c = 15 \text{ lbs.} c=15 lbs. is the correct solution.
Word Problem Example 2
| Three friends go to a local arcade and decide to split all their winnings. On their first coin, the three friends hit the jackpot on one of the games. The game dispenses an unknown amount of tickets, but after the three friends split them up evenly, each friend got 200 tickets. Create an equation to model this situation, then solve for t t t, the total amount of tickets won in the jackpot. |
Solution: Let t= the total amount of tickets won from the jackpot t = \text{ the total amount of tickets won from the jackpot} t= the total amount of tickets won from the jackpot. SInce we know the three friends split the jackpot evenly, we can model the situation with the equation:
t3=200 \dfrac{t}{3} = 200 3t=200
Then, since t3 \dfrac{t}{3} 3t represents “t divided by 3 t \text{ divided by } 3 t divided by 3”, we will use the inverse operation, multiplication, to solve.
3⋅t3=3⋅200 \textcolor{red}{3 \cdot} \dfrac{t}{3} = \color{red}{ 3 \cdot } 200 3⋅3t=3⋅200
t=600 tickets t = 600 \text{ tickets} t=600 tickets
To check your answer, simply substitute 600 600 600 in for t t t:
6003=200 \dfrac{600}{3} = 200 3600=200
200=200✓ 200 = 200 \checkmark 200=200✓
Therefore, t=600 tickets t = 600 \text{ tickets} t=600 tickets is the correct solution.